Investors have been spoiled by the trend in falling long-term interest rates over the past 40 years, and have only occasionally had to worry about equity strategies for a rising rate environment. The economic backdrop may now be changing, but investors seem hesitant to alter their investment strategies.
During the early-1990s, we were among the first researchers to investigate “equity duration”, or equities’ sensitivity to changes in interest rates. Long-term interest rates have always been a critical factor for valuation, but equity duration was a more advanced concept in that it not only looked at interest rates, but also the relationship between earnings, interest rates, and the interaction between the two.
Long-term interest rates have started to increase as the Fed extricates itself from cornering the long-term Treasury market. Yet, up to very recently, investors have been relatively hesitant to sell the longest-duration equities. Technology, venture capital, cryptocurrencies, innovation, and disruption are all long-duration strategies that performed very well as long-term interest rates fell. It seems unrealistic to expect the biggest beneficiaries of falling interest rates to outperform as interest rates rise.
What is duration?
Duration is a measure of price’s sensitivity to interest rates measured in years. For example, a 30-year zero-coupon bond has a duration of 30 years because all of the return is received in year 30 when principal is received. A 30-year coupon paying bond would have a shorter duration because coupon payments would be received during each year until the principal is returned in year 30. Currently, the 30-year coupon paying government note has a duration of about 23 years versus the 30-year duration of the zero.
Shorter duration bonds should have less interest-rate sensitivity than would longer-duration bonds. All things being equal, a bond with a 30-year duration should move up or down 30% for every one-percentage point move in interest rates. A 30-year zero coupon bond would likely outperform the coupon-paying equivalent during periods of falling interest rates, and vice versa.
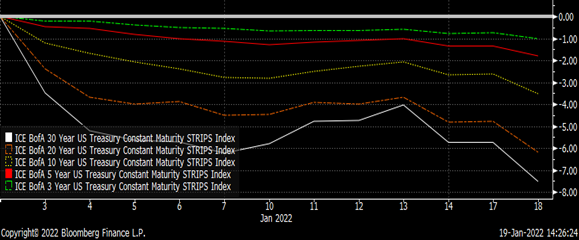
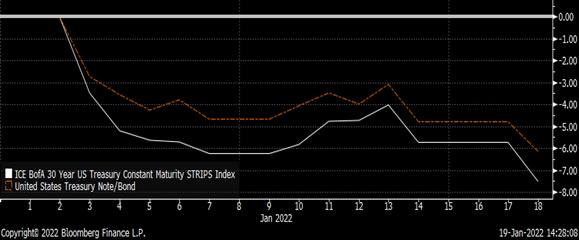
Chart 1 shows the duration effect on performance so far during 2022 of zero coupon bonds of varying maturities. Chart 2 shows a similar comparison between the 30-year zero coupon bonds and current 30-year coupon bond.
Chart 1: US Treasury Zero-Coupon Bond Performance
12/31/2021 – 1/24/2022
Chart 2: 30 Year US Treasury Zero-Coupon vs. 30 Year Treasury Bond Performance
12/31/2021 – 1/24/2022
How does duration apply to equities?
Our research during the 1990s highlighted a way to theoretically measure the interest rate sensitivity within equities. Unlike fixed-income, which as the name implies pays fixed rates of interest, equity cash flows can vary immensely and must be estimated. Analysts’ estimates for near-term and longer-term growth rates can be used to sketch expected future cash flows.
Such analyses suggest higher dividend paying stocks (like Utilities) have shorter durations because of their higher near-term dividend payments, whereas growth stocks (like Technology) have longer durations because of the lack of dividends but an anticipated long-term return.
However, investors must account for the correlation between interest rates and earnings. The earnings and cash flows of cyclical stocks are often positively correlated with interest rates because their earnings are economically sensitive. Interest rates tend to rise as the economy strengthens, but so do the earnings of cyclical stocks. Interest rates generally fall as the economy weakens, but so do the earnings of cyclical stocks.
Thus, the durations of cyclical stocks will change, sometimes significantly, as interest rates increase or decrease. Because earnings and cash flow estimates for cyclical industries tend to increase as interest rates increase, cyclical stocks’ durations will incrementally shorten as rates increase. On the other hand, cyclical earnings and cash flow estimates decrease as rates decrease. However, the earnings and cash flow estimates for stable or long-term growth companies don’t tend to change as much when interest rates change because their cash flows are relatively unaffected by the overall economy.
A simple measure of equity duration
A simple measure of equity duration is a PE ratio. A PE ratio of 5, within this simple model, suggests today’s price equates to 5 years of earnings, whereas a PE ratio of 30 suggests today’s price equates to 30 years of earnings.
A standard axiom of investing is PE ratios expand as interest rates fall and, ignoring cyclical earnings growth, a stock with a PE of 30 will tend to outperform a stock with a PE of 5 when interest rates fall just as a 30-year zero-coupon bond will outperform a 5-year zero. The reverse tends to be true when interest rates increase.
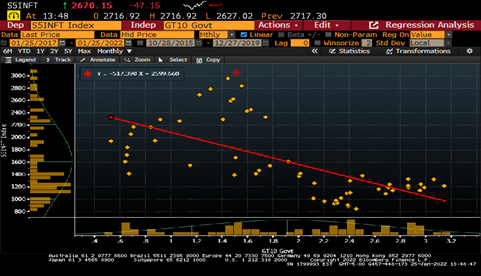
Charts 3 and 4 show the relationship between the 10-year T-bond yield and the S&P 500® Technology and S&P 500® Energy Indices over the past 5 years. Certainly interest rates are not the only variable effecting the pricing and the valuation of either sector, but the charts indicate that the longer-duration Technology sector has greater interest-rate sensitivity than does the shorter-duration Energy sector.
Chart 3: S&P 500® Technology vs. 10 Year Treasury Yield
(1/25/2017 – 1/25/2022)
Chart 4: S&P 500® Energy vs. 10-Year Treasury Yield
(1/25/2017 – 1/25/2022)
Chart 5 shows the dividend yields of S&P 500® Sectors. Only the super growth-oriented sectors, like Communication Services, Technology, and Consumer Discretionary, currently have lower dividend yields than the S&P 500®. It is highly likely that those sectors’ performances will be hurt more than the higher yielding sectors if interest rates do continue to trend upward.
Chart 5: S&P 500® Sectors by Dividend Yield
(as of 1/24/2022)
Negative convexity: Earnings estimates falling as rates rise
As mentioned, equity duration can change significantly because of the interaction between earnings and interest rates, i.e., cyclical earnings tend to accelerate during economic environments that cause interest rates to rise. In a fixed-income sense, that would be called “positive convexity”.
Currently, it appears as though earnings expectations for growth stocks might be too optimistic, and it could be that growth stock earnings decelerate as interest rates rise. That would be called “negative convexity”.
The table below, from BofA Equity and Quantitative Strategy, shows various bottom-up fundamental forecasts for economic sectors. Several points may be worth considering:
- Bottom-up estimates tend to be optimistic.
- The growth rate for the Technology sector for 2022 is only 9%, which is considerably slower than that for other sectors.
- The long-term growth rate forecast for the Technology sector presently ranks only 6th of the 11 sectors. Most investors probably think it’s either 1st or 2nd.
- The equity duration of the 2 highest p/e sectors, (i.e. perceived high “growth”) is substantially more than that of stable or cyclical ones. As such, it again suggests that these sector returns could be inferior if interest rates do continue to increase.
Table 1: BofA US Equity and Quantitative Strategy Universe Sector/Industry Factor Evaluation As of 12/31/2021
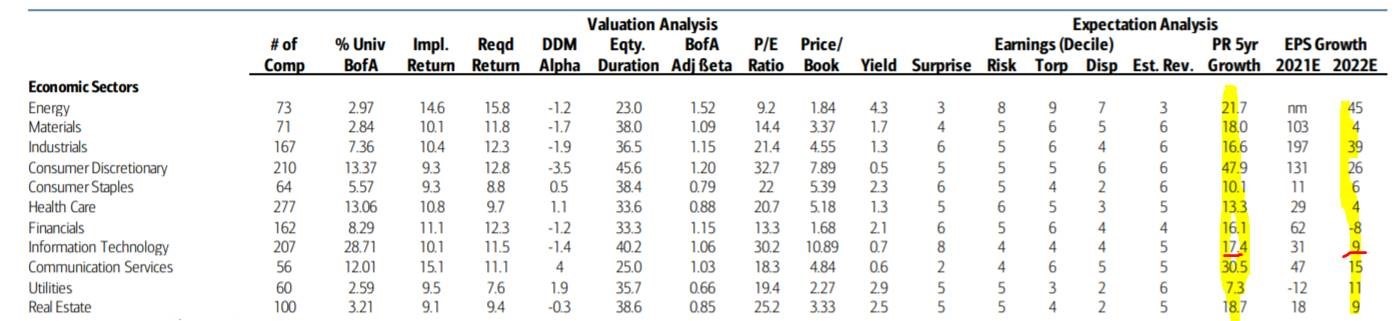
Source: BofA US Equity and Quant Strategy as published in BofA Global Research Quantitative Profiles, January 14th, 2022.
Interest rates do matter
The concept of equity duration helps to explain why certain sectors outperform or underperform during periods of rising or falling interest rates.
Investors have been spoiled by decades of falling interest rates. If that trend ends, then it seems unlikely that the long-term growth leadership will continue. Investors might consider looking at shorter-duration equity strategies to help buffer any trend in rising rates.
INDEX DESCRIPTIONS:
The following descriptions, while believed to be accurate, are in some cases abbreviated versions of more detailed or comprehensive definitions available from the sponsors or originators of the respective indices. Anyone interested in such further details is free to consult each such sponsor’s or originator’s website.
Indexes are not available for direct investment.
S&P 500®: The S&P 500® Index is an unmanaged, capitalization-weighted index designed to measure the performance of the broad US market. The index includes 500 leading companies covering approximately 80% of available market capitalization.
Sector/Industries: Sector/industry references in this report are in accordance with the Global Industry Classification Standard (GICS®) developed by MSCI Barra and S&P Global.richard
30 Year US Treasury Zero Coupon Bond: ICE® BofA US 30 Year Constant Maturity STRIP Index (S030) ICE BofA US 30 Year Constant Maturity STRIP Index tracks the performance of a single synthetic US Treasury STRIP purchased at the beginning of the month, held for one month, and then sold at the end of the month with the proceeds rolled into a new instrument. Therefore, on the purchase date, the bond has a maturity exactly equal to the stated maturity of the index, and at the point it is sold it is one month short of the index stated maturity. The synthetic STRIP has a zero coupon, a purchase yield equal to the yield of the corresponding point on the coupon STRIP curve, and a purchase price which is derived from the purchase yield. The coupon STRIP curve is fitted from the observed prices of all outstanding US Treasury coupon STRIPs. Each day thereafter, the instrument is priced by discounting its cash flow at the current day’s coupon STRIP curve, while taking account of the passage of time. At the end of the month, the security is sold and the proceeds are rolled into a new instrument with a maturity equal to the stated maturity of the index.
20 Year US Treasury Zero Coupon Bond: ICE® BofA US 20 Year Constant Maturity STRIP Index (S020) ICE BofA US 20 Year Constant Maturity STRIP Index tracks the performance of a single synthetic US Treasury STRIP purchased at the beginning of the month, held for one month, and then sold at the end of the month with the proceeds rolled into a new instrument. Therefore, on the purchase date, the bond has a maturity exactly equal to the stated maturity of the index, and at the point it is sold it is one month short of the index stated maturity. The synthetic STRIP has a zero coupon, a purchase yield equal to the yield of the corresponding point on the coupon STRIP curve, and a purchase price which is derived from the purchase yield. The coupon STRIP curve is fitted from the observed prices of all outstanding US Treasury coupon STRIPs. Each day thereafter, the instrument is priced by discounting its cash flow at the current day’s coupon STRIP curve, while taking account of the passage of time. At the end of the month, the security is sold and the proceeds are rolled into a new instrument with a maturity equal to the stated maturity of the index.
10 Year US Treasury Zero Coupon Bond: ICE® BofA US 10 Year Constant Maturity STRIP Index (S010) ICE BofA US 10 Year Constant Maturity STRIP Index tracks the performance of a single synthetic US Treasury STRIP purchased at the beginning of the month, held for one month, and then sold at the end of the month with the proceeds rolled into a new instrument. Therefore, on the purchase date, the bond has a maturity exactly equal to the stated maturity of the index, and at the point it is sold it is one month short of the index stated maturity. The synthetic STRIP has a zero coupon, a purchase yield equal to the yield of the corresponding point on the coupon STRIP curve, and a purchase price which is derived from the purchase yield. The coupon STRIP curve is fitted from the observed prices of all outstanding US Treasury coupon STRIPs. Each day thereafter, the instrument is priced by discounting its cash flow at the current day’s coupon STRIP curve, while taking account of the passage of time. At the end of the month, the security is sold and the proceeds are rolled into a new instrument with a maturity equal to the stated maturity of the index.
5 Year US Treasury Zero Coupon Bond: ICE® BofA US 5 Year Constant Maturity STRIP Index (S005) ICE BofA US 5 Year Constant Maturity STRIP Index tracks the performance of a single synthetic US Treasury STRIP purchased at the beginning of the month, held for one month, and then sold at the end of the month with the proceeds rolled into a new instrument. Therefore, on the purchase date, the bond has a maturity exactly equal to the stated maturity of the index, and at the point it is sold it is one month short of the index stated maturity. The synthetic STRIP has a zero coupon, a purchase yield equal to the yield of the corresponding point on the coupon STRIP curve, and a purchase price which is derived from the purchase yield. The coupon STRIP curve is fitted from the observed prices of all outstanding US Treasury coupon STRIPs. Each day thereafter, the instrument is priced by discounting its cash flow at the current day’s coupon STRIP curve, while taking account of the passage of time. At the end of the month, the security is sold and the proceeds are rolled into a new instrument with a maturity equal to the stated maturity of the index.
3 Year US Treasury Zero Coupon Bond: ICE® BofA US 3 Year Constant Maturity STRIP Index (S003) ICE BofA US 3 Year Constant Maturity STRIP Index tracks the performance of a single synthetic US Treasury STRIP purchased at the beginning of the month, held for one month, and then sold at the end of the month with the proceeds rolled into a new instrument. Therefore, on the purchase date, the bond has a maturity exactly equal to the stated maturity of the index, and at the point it is sold it is one month short of the index stated maturity. The synthetic STRIP has a zero coupon, a purchase yield equal to the yield of the corresponding point on the coupon STRIP curve, and a purchase price which is derived from the purchase yield. The coupon STRIP curve is fitted from the observed prices of all outstanding US Treasury coupon STRIPs. Each day thereafter, the instrument is priced by discounting its cash flow at the current day’s coupon STRIP curve, while taking account of the passage of time. At the end of the month, the security is sold and the proceeds are rolled into a new instrument with a maturity equal to the stated maturity of the index.